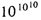Philosophy of mathematics

[Excerpted by kind permission of the author and publisher from Intuitionism: an Introduction, Arend Heyting, North-Holland, 1956 (3rd ed., 1971).]

Class: How do you do, Mr. Int? Did you not flee the town on this fine summer day?

Int: I had some ideas and worked them out at the library.

Class: Industrious bee! How are you getting along?

Int: Quite well. Shall we have a drink?

Class: Thank you. I bet you worked on that hobby of yours, rejection of the excluded middle, and the rest. I never understood why logic should be reliable everywhere else, but not in mathematics.

Int: We have spoken about that subject before. The idea that for the description of some kinds of objects another logic may be more adequate than the customary one has sometimes been discussed. But it was Brouwer who first discovered an object which actually requires a different form of logic, namely the mental mathematical construction [L.E.J. Brouwer 1908]. The reason is that in mathematics from the very beginning we deal with the infinite, whereas ordinary logic is made for reasoning about finite collections.

Class: I know, but in my eyes logic is universal and applies to the infinite as well as to the finite.

Int: You ought to consider what Brouwer's program was [L. E. J. Brouwer 1907]. It consisted in the investigation of mental mathematical construction as such, without reference to questions regarding the nature of the constructed objects, such as whether these objects exist independently of our knowledge of them. That this point of view leads immediately to the rejection of the principle of excluded middle, I can best demonstrate by an example.

Let us compare two definitions of natural numbers, say k and l.

• k is the greatest prime such that k - 1 is also a prime, or k = 1 if such a number does not exist.
• l is the greatest prime such that l - 2 is also a prime, or l = 1 if such a number does not exist.

Classical mathematics neglects altogether the obvious difference in character between these definitions. k can actually be calculated (k = 3), whereas we possess no method for calculating l, as it is not known whether the sequence of pairs of twin primes p, p + 2 is finite or not. Therefore intuitionists reject II as a definition of an integer; they consider an integer to be well-defined only if a method for calculating it is given. Now this line of thought leads to the rejection of the principle of excluded middle, for if the sequence of twin primes were either finite or not finite, II would define an integer.

Class: One may object that the extent of our knowledge about the existence or non-existence of a last pair of twin primes is purely contingent and entirely irrelevant in questions of mathematical truth. Either an infinity of such pairs exist, in which case l = 1; or their number is finite, in which case l equals the greatest prime such that l - 2 is also a prime. In every conceivable case l is defined; what does it matter whether or not we can actually calculate the number?

Int: Your argument is metaphysical in nature. If "to exist" does not mean "to be constructed", it must have some metaphysical meaning. It cannot be the task of mathematics to investigate this meaning or to decide whether it is tenable or not. We have no objection against a mathematician privately admitting any metaphysical theory he likes, but Brouwer's program entails that we study mathematics as something simpler, more immediate than metaphysics. In the study of mental mathematical constructions "to exist" must be synonymous with "to be constructed".

Class: That is to say, as long as we do not know if there exists a last pair of twin primes, II is not a definition of an integer, but as soon as this problem is solved, it suddenly becomes such a definition. Suppose on January 1, 1970 it is proved that an infinity of twin primes exists; from that moment l = 1. Was l = 1 before that date or not? [Menger 1930].

Int: A mathematical assertion affirms the fact that a certain mathematical construction has been effected. It is clear that before the construction was made, it had not been made. Applying this remark to your example, we see that before Jan. 1, 1970 it had not been proved that l = 1. But this is not what you mean. It seems to me that in order to clarify the sense of your question you must again refer to metaphysical concepts: to some world of mathematical things existing independently of our knowledge, where "l = 1" is true in some absolute sense. But I repeat that mathematics ought not to depend upon such notions as these. In fact all mathematicians and even intuitionists are convinced that in some sense mathematics bear upon eternal truths, but when trying to define precisely this sense, one gets entangled in a maze of metaphysical difficulties. The only way to avoid them is to banish them from mathematics. This is what I meant by saying that we study mathematical constructions as such and that for this study classical logic is inadequate.

Class: Here come our friends Form and Letter. Boys, we are having a most interesting discussion on intuitionism.

Letter: Could you speak about anything else with good old Int? He is completely submerged in it.

Int: Once you have been struck with the beauty of a subject, devote your life to it!

Form: Quite so! Only I wonder how there can be beauty in so indefinite a thing as intuitionism. None of your terms are well-defined, nor do you give exact rules of derivation. Thus one for ever remains in doubt as to which reasonings are correct and which are not [R. Carnap 1934b, p. 41; 1937, p. 46; W. Dubislav 1932, pp. 57, 75]. In daily speech no word has a perfectly fixed meaning; there is always some amount of free play, the greater, the more abstract the notion is. This makes people miss each other's point, also in non-formalized mathematical reasonings. The only way to achieve absolute rigour is to abstract all meaning from the mathematical statements and to consider them for their own sake, as sequences of signs, neglecting the sense they may convey. Then it is possible to formulate definite rules for deducing new statements from those already known and to avoid the uncertainty resulting from the ambiguity of language.

Int: I see the difference between formalists and intuitionists mainly as one of taste. You also use meaningful reasoning in what Hilbert called metamathematics, but your purpose is to separate these reasonings from purely formal mathematics and to confine yourself to the most simple reasonings possible. We, on the contrary, are interested not in the formal side of mathematics, but exactly in that type of reasoning which appears in metamathematics; we try to develop it to its farthest consequences. This preference arises from the conviction that we find here one of the most fundamental faculties of the human mind.

Form: If you will not quarrel with formalism, neither will I with intuitionism. Formalists are among the most pacific of mankind. Any theory may be formalized and then becomes subject to our methods. Also intuitionistic mathematics may and will be thus treated [R. Carnap 1934b, p. 44; 1937, p. 51].

Class: That is to say, intuitionistic mathematics ought to be studied as a part of mathematics. In mathematics we investigate the consequences of given assumptions; the intuitionistic assumptions may be interesting, but they have no right to a monopoly.

Int: Nor do we claim that; we are content if you admit the good right of our conception. But I must protest against the assertion that intuitionism starts from definite, more or less arbitrary assumptions. Its subject, constructive mathematical thought, determines uniquely its premises and places it beside, not interior to, classical mathematics, which studies another subject, whatever subject that may be. For this reason an agreement between formalism and intuitionism by means of the formalization of intuitionistic mathematics is also impossible. It is true that even in intuitionistic mathematics the finished part of a theory may be formalized. It will be useful to reflect for a moment upon the meaning of such a formalization. We may consider the formal system as the linguistic expression, in a particularly suitable language, of mathematical thought.

If we adopt this point of view, we clash against the obstacle of the fundamental ambiguousness of language. As the meaning of a word can never be fixed precisely enough to exclude every possibility of misunderstanding, we can never be mathematically sure that the formal system expresses correctly our mathematical thoughts.

However, let us take another point of view. We may consider the formal system itself as an extremely simple mathematical structure; its entities (the signs of the system) are associated with other, often very complicated, mathematical structures. In this way formalizations may be carried out inside mathematics, and it becomes a powerful mathematical tool. Of course, one is never sure that the formal system represents fully any domain of mathematical thought; at any moment the discovering of new methods of reasoning may force us to extend the formal system.

Form: For several years we have been familiar with this situation. Gödel's incompleteness theorem showed us that any consistent formal system of number-theory may be extended consistently in different ways.

Int: The difference is that intuitionism proceeds independently of the formalization, which can but follow after the mathematical construction.

Class: What puzzles me most is that you both seem to start from nothing at all. You seem to be building castles in the air. How can you know if your reasoning is sound if you do not have at your disposal the infallible criterion given by logic? Yesterday I talked with Sign, who is still more of a relativist than either of you. He is so slippery that no argument gets hold of him, and he never comes to any somewhat solid conclusion. I fear this fate for anybody who discards the support of logic, that is, of common sense.

Sign: Speak of the devil and his imp appears. Were you speaking ill of me?

Class: I alluded to yesterday's discussion. To-day I am attacking these other two damned relativists.

Sign: I should like to join you in that job, but first let us hear the reply of your opponents. Please meet my friend Prag; he will be interested in the discussion.

Form: How do you do? Are you also a philosopher of science?

Prag: I hate metaphysics.

Int: Welcome, brother!

Form: Why, I would rather not defend my own position at the moment, as our discussion has dealt mainly with intuitionism and we might easily confuse it. But I fear that you are wrong as to intuitionistic logic. It has indeed been formalized and valuable work in this field has been done by a score of authors. This seems to prove that intuitionists esteem logic more highly than you think, though it is another logic than you are accustomed to.

Int: I regret to disappoint you. Logic is not the ground upon which I stand. How could it be? It would in turn need a foundation, which would involve principles much more intricate and less direct than those of mathematics itself. A mathematical construction ought to be so immediate to the mind and its result so clear that it needs no foundation whatsoever. One may very well know whether a reasoning is sound without using any logic; a clear scientific conscience suffices. Yet it is true that intuitionistic logic has been developed. To indicate what its significance is, let me give you an illustration. Let A designate the property of an integer of being divisible by 8, B the same by 4, C the same by 2. For 8a we may write 4 ´ 2a; by this mathematical construction P we see that the property A entails B (A ® B). A similar construction Q shows B ® C. By effecting first P, then Q (juxtaposition of P and Q) we obtain 8a = 2 ´ (2 ´ 2a) showing A ® C. This process remains valid if for A, B, C we substitute arbitrary properties: If the construction P shows that A ® B and Q shows that B ® C, then the juxtaposition of P and Q shows that A ® C. We have obtained a logical theorem. The process by which it is deduced shows us that it does not differ essentially from mathematical theorems; it is only more general, e.g., in the same sense that "addition of integers is commutative" is a more general statement than "2+3=3+2". This is the case for every logical theorem: it is but a mathematical theorem of extreme generality; that is to say, logic is a part of mathematics, and can by no means serve as a foundation for it. At least, this is the conception of logic to which I am naturally led; it may be possible and desirable to develop other forms of logic for other purposes.

It is the mathematical logic which I just described that has been formalized. The resulting formal system proves to have peculiar properties, very interesting when compared to those of other systems of formal logic. This fact has led to the investigations to which Mr. Form alluded, but, however interesting, they are tied but very loosely to intuitionistic mathematics.

Letter: In my opinion all these difficulties are imaginary or artificial. Mathematics is quite a simple thing. I define some signs and I give some rules for combining them; that is all.

Form: You want some modes of reasoning to prove the consistency of your formal system.

Letter: Why should I want to prove it? You must not forget that our formal systems are constructed with the aim towards applications and that in general they prove useful; this fact would be difficult to explain if every formula were deducible in them. Thereby we get a practical conviction of consistency which suffices for our work. What I contest in intuitionism is the opinion that mathematics has anything to do with the infinite. I can write down a sign, say a, and call it the cardinal number of the integers. After that I can fix rules for its manipulation in agreement with those which Mr. Class uses for this notion; but in doing this I operate entirely in the finite. As soon as the notion of infinity plays a part, obscurity and confusion penetrate into the reasoning. Thus all the intuitionistic assertions about the infinite seem to me highly ambiguous, and it is even questionable whether such a sign as 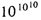has any other meaning than as a figure on paper with which we operate according to certain rules [J. Dieudonné 1951].

Int: Of course your extreme finitism grants the maximum of security against misunderstanding, but in our eyes it implies a denial of understanding which it is difficult to accept. Children in the elementary school understand what the natural numbers are and they accept the fact that the sequence of natural numbers can be indefinitely continued.

Letter: It is suggested to them that they understand.

Int: That is no objection, for every communication by means of language may be interpreted as suggestion. Also Euclid in the 20th proposition of Book IX, where he proved that the set of prime numbers is infinite, knew what he spoke about. This elementary notion of natural numbers, familiar to every thinking creature, is fundamental in intuitionistic mathematics. We do not claim for it any form of certainty or definiteness in an absolute sense, which would be unrealizable, but we content that it is sufficiently clear to build mathematics upon.

Letter: My objection is that you do not suppose too little, as Mr. Class thinks, but far too much. You start from certain principles which you take as intuitively clear without any explanation and you reject other modes of reasoning without giving any grounds for that discrimination. For instance, to most people the principle of the excluded middle seems at least as evident as that of complete induction. Why do you reject the former and accept the latter? Such an unmotivated choice of first principles gives to your system a strongly dogmatic character.

Int: Indeed intuitionistic assertions must seem dogmatic to those who read them as assertions about facts, but they are not meant in this sense. Intuitionistic mathematics consists, as I have explained already to Mr. Class, in mental constructions; a mathematical theorem expresses a purely empirical fact, namely the success of a certain construction. "2+2=3+1" must be read as an abbreviation for the statement: "I have effected the mental constructions indicated by "2+2" and by "3+1" and I have found that they lead to the same result." Now tell me where the dogmatic element can come in; not in the mental construction itself, as is clear by its very nature as an activity, but no more in the statements made about the constructions, for they express purely empirical results.

Letter: Yet you contend that these mental constructions lead to some sort of truth; they are not a game of solitaire, but in some sense must be of value for mankind, or you would be wrong in annoying others with them. It is in this pretence that I see the dogmatic element. The mathematical intuition inspires you with objective and eternal truths; in this sense your point of view is not only dogmatic, but even theological [H.B. Curry 1951, p. 6].

Int: In the first instance, my mathematical thoughts belong to my individual intellectual life and are confined to my personal mind, as is the case for other thoughts as well. We are generally convinced that other people have thoughts analogous to our own and that they can understand us when we express our thoughts in words, but we also know that we are never quite sure of being faultlessly understood. In this respect, mathematics does not essentially differ from other subjects; if for this reason you consider mathematics to be dogmatic, you ought to call any human reasoning dogmatic. The characteristic of mathematical thought is, that it does not convey truth about the external world, but is only concerned with mental constructions. Now we must distinguish between the simple practice of mathematics and its valuation. In order to construct mathematical theories no philosophical preliminaries are needed, but the value we attribute to this activity will depend upon our philosophical ideas.

Sign: In the way you treat language you put the clock back. Primitive language has this floating, unsteady character you describe, and the language of daily life is still in the main of the same sort, but as soon as scientific thought begins, the formalization of language sets in. In the last decades significists have studied this process. It has not yet come to an end, for more strictly formalized languages are still being formed.

Int: If really the formalization of language is the trend of science, then intuitionistic mathematics does not belong to science in this sense of the word. It is rather a phenomenon of life, a natural activity of man, which itself is open to study by scientific methods; it has actually been studied by such methods, namely that of formalizing intuitionistic reasoning and the signific method, but it is obvious that this study does not belong to intuitionistic mathematics, nor do its results. That such a scientific examination of intuitionistic mathematics will never produce a complete and definite description of it, no more than a complete theory of other phenomena is attainable, is clearly to be seen. Helpful and interesting as these metaintuitionistic considerations may be, they cannot be incorporated into intuitionistic mathematics itself. Of course, these remarks do not apply to formalization inside mathematics, as I described it a few moments ago.

Prag: Allow me to underline what Mr. Sign said just now. Science proceeds by formalization of language; it uses this method because it is efficient. In particular the modern completely formalized languages have appeared to be most useful. The ideal of the modern scientist is to prepare an arsenal of formal systems ready for use from which he can choose, for any theory, that system which correctly represents the experimental results. Formal systems ought to be judged by this criterion of usefulness and not by a vague and arbitrary interpretation, which is preferred for dogmatic or metaphysical reasons.

Int: It seems quite reasonable to judge a mathematical system by its usefulness. I admit that from this point of view intuitionism has as yet little chance of being accepted, for it would be premature to stress the few weak indications that it might be of some use in physics [J.L. Destouches 1951]; in my eyes its chances of being useful for philosophy, history and the social sciences are better. In fact, mathematics, from the intuitionistic point of view, is a study of certain functions of the human mind, and as such it is akin to these sciences. But is usefulness really the only measure of value? It is easy to mention of score of valuable activities which in no way support science, such as the arts, sports, and light entertainment. We claim for intuitionism a value of this sort, which it is difficult to define beforehand, but which is clearly felt in dealing with the matter. You know how philosophers struggle with the problem of defining the concept of value in art; yet every educated person feels this value. The case is analogous for the value of intuitionistic mathematics.

Form: For most mathematicians this value is affected fatally by the fact that you destroy the most precious mathematical results; a valuable method for the foundation of mathematics ought to save as much as possible of its results [D. Hilbert 1922]. This might even succeed by constructive methods; for definitions of constructiveness other than that advocated by the intuitionists are conceivable. For that matter, even the small number of actual intuitionists do not completely agree about the delimitation of the constructive. The most striking example is the rejection by Griss of the notion of negation, which other intuitionists accept as perfectly clear [H. Freudenthal 1936; G.F.C. Griss 1946a, p. 24; 1946b]. It seems probable, on the other hand, that a somewhat more liberal conception of the constructive might lead to the saving of the vital parts of classical mathematics.

Int: As intuitionists speak a non-formalized language, slight divergences of opinion between them can be expected. Though they have arisen sooner and in more acute forms than we could foresee, they are in no way alarming, for they all concern minor points and do not affect the fundamental ideas, about which there is complete agreement. Thus it is most unlikely that a wider conception of constructiveness could obtain the support of intuitionists. As to the mutilation of mathematics of which you accuse me, it must be taken as an inevitable consequence of our standpoint. It can also be seen as the excision of noxious ornaments, beautiful in form, but hollow in substance, and it is at least partly compensated for by the charm of subtle distinctions and witty methods by which intuitionists have enriched mathematical thought.

Form: Our discussion has assumed the form of a discussion of values. I gather from your words that you are ready to acknowledge the value of other conceptions of mathematics, but that you claim for your conception a value of its own. Is that right?

Int: Indeed, the only positive contention in the foundation of mathematics which I oppose is that classical mathematics has a clear sense; I must confess that I do not understand that. But even those who maintain that they do understand it might still be able to grasp our point of view and to value our work.

Letter: It is shown by the paradoxes that classical mathematics is not perfectly clear.

Form: Yes, but intuitionistic criticism goes much farther than is necessary to avoid the paradoxes; Mr. Int has not even mentioned them as an argument for his conception, and no doubt in his eyes consistency is but a welcome by-product of intuitionism.

Sign: You describe your activity as mental construction, Mr. Int, but mental processes are only observable through the acts to which they lead, in your case through the words you speak and the formulas you write. Does not this mean that the only way to study intuitionism is to study the formal system which it constructs?

Int: When looking at the tree over there, I am convinced I see a tree, and it costs considerable training to replace this conviction by the knowledge that in reality lightwaves reach my eyes, leading me to the construction of an image of the tree. In the same way, in speaking to you I am convinced that I press my opinions upon you, but you instruct me that in reality I produce vibrations in the air, which cause you to perform some action, e.g. to produce other vibrations. In both cases the first view is the natural one, the second is a theoretical construction. It is too often forgotten that the truth of such constructions depends upon the present state of science and that the words "in reality" ought to be translated into "according to the contemporary view of scientists". Therefore I prefer to adhere to the idea that, when describing intuitionistic mathematics, I convey thoughts to my hearers; these words ought to be taken not in the sense of some philosophical system, but in the sense of every-day life.

Sign: Then intuitionism, as a form of interaction between men, is a social phenomenon and its study belongs to the history of civilization.

Int: Its study, not its practice. Here I agree with Mr. Prag: primum vivere, deinde philosophari, and if we like we can leave the latter to others. Let those who come after me wonder why I built up these mental constructions and how they can be interpreted in some philosophy; I am content to build them in the conviction that in some way they will contribute to the clarification of human thought.

Prag: It is a common fault of philosophers to speak about things they know but imperfectly and we are near to being caught in that trap. Is Mr. Int willing to give us some samples of intuitionistic reasoning, in order that we may better be able to judge the quality of the stuff?

Int: Certainly, and even I am convinced that a few lessons will give you a better insight into it than lengthy discussions. May I beg those gentlemen who are interested in my explanations, to follow me to my classroom?