Resources Math 3 Course Home Page Math 3 Course Syllabus Practice Exams Textbook Home Page Post a Comment

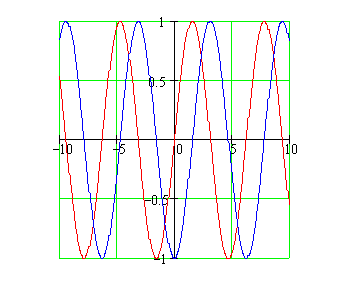
Contents In this lecture we review (or introduce) those aspects of the trigonometric functions that we will need for the study of calculus. The trig functions are particularly useful for modeling periodic behavior. Quick Question What is the trig identity for sin(x − π/2) illustrated in the following graph? Answer Outline Outline for Trigonometric Functions Textbook Trigonometric Functions Today's Homework Log into WebWorK Quiz Trigonometric Functions Quiz Examples Evaluate a quotient of two trig expressions exactly A particle moving back and forth on the x axis has its position given as a function of time t. Where is the particle when t = 0 and how long does it take to return to this position? Find all solutions to the equation 1 + 2sin(x) − (sin(x))2 = (cos(x))2 − 1 Applets Definitions of sin(x) and cos(x) Trigonometric Identities Videos Tricks to translate and scale sin(x) Graph cos(5(x – π/2)) Graph 3sin(2x) Basic trig

Lecture 2 | Index | Lecture 4