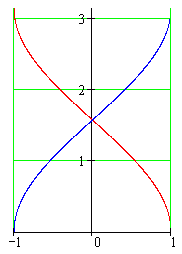
Find the area of the hourglass-shaped area between the curves arcsin(x) + π/2 and arccos(x) and the lines y = 0 and y = π.

To make it easier to integrate, we can integrate with respect to y. So we can make x the subject for each curve:

For the first curve, making x the subject, x = sin(y + π/2) = cos(y). We can then set up the following integral:

For the second curve, making x the subject, x = cos(y), which is the exact same equation obtained above. Therefore the area is also 2

The total area equals 4.