
April 7, 1997
1
Discussion of geometry problems from Wednesday. Especially relative distance of sun and
moon. What were the suspicious parts? Determination of angle. Actual measurement was in terms of
hours. Also tables of sine and cosine were not accurate. How do we determine these now? How did
people used to do it? Note comment at end of p26. One of the things you notice in C is tables of
sine and cosine. By the way, this is just a little taste of Copernicus--have a look at the full
volume of his stuff in the library.
2
- a. For the purposes of describing retrograde motion qualitatively, which is simpler,
the Ptolemaic model or the Copernican model? Why? Based on Friday's exercise.
- b. Which is more satisfying? Why?
3
Discuss reading from Copernicus. How does it compare to Euclid? Note p. 315, how he takes
specific angles to do his computation. Variables were recently invented and not in common use. And
they usually stood in for integers, not angles.
4
Sample problem, Copernicus p. 318. Picture on board of Mercury around sun. What does C.
mean by ecliptic? eccentric? How does C. set up problem? He points out that highest latitudes of
venus occur at farthest points from sun. He hopes to explain this by a construction.
5
From "Revolutions"
P.318
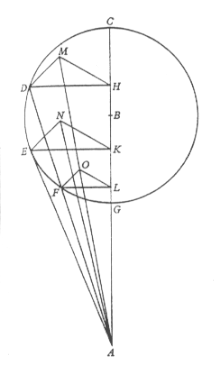
3-D image of problem. Try to see similar and congruent triangles. Note the use of
minimizing argument.
6
Why is this argument a triumph for C? You can't make this argument in Ptolemaic system
because sun's position isn't inherently linked to venus's. This argument is a vindication of Euclid
as having the right world view.
7
For next time: study the same readings (C and E) for stylistic parallels. How are
arguments constructed?
| <- Prev
| Next -> |




