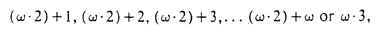
Selected readings
SECOND EDITION
On the infinite
As a result of his penetrating critique, Weierstrass has provided a solid foundation for mathematical analysis. By elucidating many notions, in particular those of minimum, function, and differential quotient, he removed the defects which were still found in the infinitesimal calculus, rid it of all confused notions about the infinitesimal, and thereby completely removed the difficulties which stem from that concept. If in analysis today there is complete agreement and certitude in employing the deductive methods which are based on the concepts of irrational number and limit, and if in even the most complex questions of the theory of differential and integral equations, notwithstanding the use of the most ingenious and varied combinations of the different kinds of limits, there nevertheless is unanimity with respect to the results obtained, then this happy state of affairs is due primarily to Weierstrass's scientific work.
And yet in spite of the foundation Weierstrass has provided for the infinitesimal calculus, disputes about the foundations of analysis still go on.
These disputes have not terminated because the meaning of the infinite, as that concept is used in mathematics, has never been completely clarified. Weierstrass's analysis did indeed eliminate the infinitely large and the infinitely small by reducing statements about them to [statements about] relations between finite magnitudes. Nevertheless the infinite still appears in the infinite numerical series which defines the real numbers and in the concept of the real number system which is thought of as a completed totality existing all at once.
In his foundation for analysis, Weierstrass accepted unreservedly and used repeatedly those forms of logical deduction in which the concept of the infinite comes into play, as when one treats of all real numbers with a certain property or when one argues that there exist real numbers with a certain property.
Hence the infinite can reappear in another guise in Weierstrass's theory and thus escape the precision imposed by his critique. It is, therefore, the problem of the infinite in the sense just indicated which we need to resolve once and for all. Just as in the limit processes of the infinitesimal calculus, the infinite in the sense of the infinitely large and the infinitely small proved to be merely a figure of speech, so too we must realize that the infinite in the sense of an infinite totality, where we still find it used in deductive methods, is an illusion. Just as operations with the infinitely small were replaced by operations with the finite which yielded exactly the same results and led to exactly the same elegant formal relationships, so in general must deductive methods based on the infinite be replaced by finite procedures which yield exactly the same results; i.e., which make possible the same chains of proofs and the same methods of getting formulas and theorems.
The goal of my theory is to establish once and for all the certitude of mathematical methods. This is a task which was not accomplished even during the critical period of the infinitesimal calculus. This theory should thus complete what Weierstrass hoped to achieve by his foundation for analysis and toward the accomplishment of which he has taken a necessary and important step.
But a still more general perspective is relevant for clarifying the concept of the infinite. A careful reader will find that the literature of mathematics is glutted with inanities and absurdities which have had their source in the infinite. For example, we find writers insisting, as though it were a restrictive condition, that in rigorous mathematics only a finite number of deductions are admissible in a proof — as if someone had succeeded in making an infinite number of them.
Also old objections which we supposed long abandoned still reappear in different forms. For example, the following recently appeared: Although it may be possible to introduce a concept without risk, i.e., without getting contradictions, and even though one can prove that its introduction causes no contradictions to arise, still the introduction of the concept is not thereby justified. Is not this exactly the same objection which was once brought against complex-imaginary numbers when it was said: "True, their use doesn't lead to contradictions. Nevertheless their introduction is unwarranted, for imaginary magnitudes do not exist"? If, apart from proving consistency, the question of the justification of a measure is to have any meaning, it can consist only in ascertaining whether the measure is accompanied by commensurate success. Such success is in fact essential, for in mathematics as elsewhere success is the supreme court to whose decisions everyone submits.
As some people see ghosts, another writer seems to see contradictions even where no statements whatsoever have been made, viz., in the concrete world of sensation, the "consistent functioning" of which he takes as special assumption. I myself have always supposed that only statements, and hypotheses insofar as they lead through deductions to statements, could contradict one another. The view that facts and events could themselves be in contradiction seems to me to be a prime example of careless thinking.
The foregoing remarks are intended only to establish the fact that the definitive clarification of the nature of the infinite, instead of pertaining just to the sphere of specialized scientific interests, is needed for the dignity of the human intellect itself.
From time immemorial, the infinite has stirred men's emotions more than any other question. Hardly any other idea has stimulated the mind so fruitfully. Yet, no other concept needs clarification more than it does.
Before turning to the task of clarifying the nature of the infinite, we should first note briefly what meaning is actually given to the infinite. First let us see what we can learn from physics. One's first naïve impression of natural events and of matter is one of permanency, of continuity. When we consider a piece of metal or a volume of liquid, we get the impression that they are unlimitedly divisible, that their smallest parts exhibit the same properties that the whole does. But wherever the methods of investigating the physics of matter have been sufficiently refined, scientists have met divisibility boundaries which do not result from the shortcomings of their efforts but from the very nature of things. Consequently we could even interpret the tendency of modern science as emancipation from the infinitely small. Instead of the old principle natura non facit saltus, we might even assert the opposite, viz., "nature makes jumps."
It is common knowledge that all matter is composed of tiny building blocks called "atoms," the combinations and connections of which produce all the variety of macroscopic objects. Still physics did not stop at the atomism of matter. At the end of the last century there appeared the atomism of electricity which seems much more bizarre at first sight. Electricity, which until then had been thought of as a fluid and was considered the model of a continuously active agent, was then shown to be built up of positive and negative electrons.
In addition to matter and electricity, there is one other entity in physics for which the law of conservation holds, viz., energy. But it has been established that even energy does not unconditionally admit of infinite divisibility. Planck has discovered quanta of energy.
Hence, a homogeneous continuum which admits of the sort of divisibility needed to realize the infinitely small is nowhere to be found in reality. The infinite divisibility of a continuum is an operation which exists only in thought. It is merely an idea which is in fact impugned by the results of our observations of nature and of our physical and chemical experiments.
The second place where we encounter the question of whether the infinite is found in nature is in the consideration of the universe as a whole. Here we must consider the expanse of the universe to determine whether it embraces anything infinitely large. But here again modern science, in particular astronomy, has reopened the question and is endeavoring to solve it, not by the defective means of metaphysical speculation, but by reasons which are based on experiment and on the application of the laws of nature. Here, too, serious objections against infinity have been found. Euclidean geometry necessarily leads to the postulate that space is infinite. Although euclidean geometry is indeed a consistent conceptual system, it does not thereby follow that euclidean geometry actually holds in reality. Whether or not real space is euclidean can be determined only through observation and experiment. The attempt to prove the infinity of space by pure speculation contains gross errors. From the fact that outside a certain portion of space there is always more space, it follows only that space is unbounded, not that it is infinite. Unboundedness and finiteness are compatible. In so-called elliptical geometry, mathematical investigation furnishes the natural model of a finite universe. Today the abandonment of euclidean geometry is no longer merely a mathematical or philosophical speculation but is suggested by considerations which originally had nothing to do with the question of the finiteness of the universe. Einstein has shown that euclidean geometry must be abandoned. On the basis of his gravitational theory, he deals with cosmological questions and shows that a finite universe is possible. Moreover, all the results of astronomy are perfectly compatible with the postulate that the universe is elliptical.
We have established that the universe is finite in two respects, i.e., as regards the infinitely small and the infinitely large. But it may still be the case that the infinite occupies a justified place in our thinking, that it plays the role of an indispensable concept. Let us see what the situation is in mathematics. Let us first interrogate that purest and simplest offspring of the human mind, viz., number theory. Consider one formula out of the rich variety of elementary formulas of number theory, e.g., the formula
12 + 22 + 32 ... + n2 = 1/6 n (n + 1) (2n + 1)
Since we may substitute any integer whatsoever for n, for example n = 2 or n = 5, this formula implicitly contains infinitely many propositions. This characteristic is essential to a formula. It enables the formula to represent the solution of an arithmetical problem and necessitates a special idea for its proof. On the other hand, the individual numerical equations
can be verified simply by calculation and hence individually are of no especial interest.
We encounter a completely different and quite unique conception of the notion of infinity in the important and fruitful method of ideal elements. The method of ideal elements is used even in elementary plane geometry. The points and straight lines of the plane originally are real, actually existent objects. One of the axioms that hold for them is the axiom of connection: one and only one straight line passes through two points. It follows from this axiom that two straight lines intersect at most at one point. There is no theorem that two straight lines always intersect at some point, however, for the two straight lines might well be parallel. Still we know that by introducing ideal elements, viz., infinitely long lines and points at infinity, we can make the theorem that two straight lines always intersect at one and only one point come out universally true. These ideal "infinite" elements have the advantage of making the system of connection laws as simple and perspicuous as possible. Moreover, because of the symmetry between a point and a straight line, there results the very fruitful principle of duality for geometry.
Another example of the use of ideal elements are the familiar complex-imaginary magnitudes of algebra which serve to simplify theorems about the existence and number of the roots of an equation.
Just as infinitely many straight lines, viz., those parallel to each other, are used to define an ideal point in geometry, so certain systems of infinitely many numbers are used to define an ideal number. This application of the principle of ideal elements is the most ingenious of all. If we apply this principle systematically throughout an algebra, we obtain exactly the same simple and familiar laws of division which hold for the familiar whole numbers 1, 2, 3, 4, . . . . We are already in the domain of higher arithmetic.
We now come to the most aesthetic and delicately erected structure of mathematics, viz., analysis. You already know that infinity plays the leading role in analysis. In a certain sense, mathematical analysis is a symphony of the infinite.
But analysis alone does not provide us with the deepest insight into the nature of the infinite. This insight is procured for us by a discipline which comes closer to a general philosophical way of thinking and which was designed to cast new light on the whole complex of questions about the infinite. This discipline, created by George Cantor, is set theory. In this paper, we are interested only in that unique and original part of set theory which forms the central core of Cantor's doctrine, viz., the theory of transfinite numbers. This theory is, I think, the finest product of mathematical genius and one of the supreme achievements of purely intellectual human activity. What, then, is this theory?
Someone who wished to characterize briefly the new conception of the infinite which Cantor introduced might say that in analysis we deal with the infinitely large and the infinitely small only as limiting concepts, as something becoming, happening, i.e., with the potential infinite. But this is not the true infinite. We meet the true infinite when we regard the totality of numbers 1, 2, 3, 4, . . . itself as a completed unity, or when we regard the points of an interval as a totality of things which exists all at once. This kind of infinity is known as actual infinity.
Frege and Dedekind, the two mathematicians most celebrated for their work in the foundations of mathematics, independently of each other used the actual infinite to provide a foundation for arithmetic which was independent of both intuition and experience. This foundation was based solely on pure logic and made use only of deductions that were purely logical. Dedekind even went so far as not to take the notion of finite number from intuition but to derive it logically by employing the concept of an infinite set. But is was Cantor who systematically developed the concept of the actual infinite. Consider the two examples of the infinite already mentioned
The tremendous progress made in the infinitesimal calculus results mainly from operating with mathematical systems of infinitely many elements. But, as it seemed very plausible to identify the infinite with the "very large", there soon arose inconsistencies which were known in part to the ancient sophists, viz., the so-called paradoxes of the infinitesimal calculus. But the recognition that many theorems which hold for the finite (for example, the part is smaller than the whole, the existence of a minimum and a maximum, the interchangeability of the order of the terms of a sum or a product) cannot be immediately and unrestrictedly extended to the infinite, marked fundamental progress. I said at the beginning of this paper that these questions have been completely clarified, notably through Weierstrass's acuity. Today, analysis is not only infallible within its domain but has become a practical instrument for using the infinite.
It is quite natural to treat these examples from the point of view of their size. But such a treatment reveals amazing results with which every mathematician today is familiar. For when we consider the set of all rational numbers, i.e., the fractions 1/2, 1/3, 2/3, 1/4, . . ., 3/7, . . ., we notice that — from the sole standpoint of its size — this set is no larger than the set of integers. Hence we say that the rational numbers can be counted in the usual way; i.e., that they are enumerable. The same holds for the set of all roots of numbers, indeed even for the set of all algebraic numbers. The second example is analogous to the first. Surprisingly enough, the set of all the points of a square or cube is no larger than the set of points of the interval 0 to 1. Similarly for the set of all continuous functions. On learning these facts for the first time, you might think that from the point of view of size there is only one unique infinite. No, indeed! The sets in examples (1) and (2) are not, as we say, "equivalent". Rather, the set (2) cannot be enumerated, for it is larger than the set (1). We meet what is new and characteristic in Cantor's theory at this point. The points of an interval cannot be counted in the usual way, i.e., by counting 1, 2, 3, . . . . But, since we admit the actual infinite, we are not obliged to stop here. When we have counted 1, 2, 3, . . ., we can regard the objects thus enumerated as an infinite set existing all at once in a particular order. If, following Cantor, we call the type of this order W, then counting continues naturally with W + 1, W + 2, . . . up to W + W or W · 2, and then again
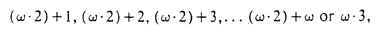
and further

so that we finally get this table:
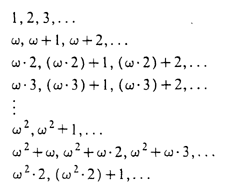
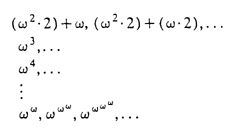
These are Cantor's first transfinite numbers, or, as he called them, the numbers of the second number class. We arrive at them simply by extending counting beyond the ordinarily enumerably infinite, i.e., by a natural and uniquely determined consistent continuation of ordinary finite counting. As until now we counted only the first, second, third, . . . member of a set, we not count also the wth, (w + 1)st, . . ., wwth member.
Given these developments one naturally wonders whether or not, by using these transfinite numbers, one can really count those sets which cannot be counted in the ordinary way.
On the basis of these concepts, Cantor developed the theory of transfinite numbers quite successfully and invented a full calculus for them. Thus, thanks to the Herculean collaboration of Frege, Dedekind, and Cantor, the infinite was made king and enjoyed a reign of great triumph. In daring flight, the infinite had reached a dizzy pinnacle of success.
But reaction was not lacking. It took in fact a very dramatic form. It set in perfectly analogously to the way reaction had set in against the development of the infinitesimal calculus. In the joy of discovering new and important results, mathematicians paid too little attention to the validity of their deductive methods. For, simply as a result of employing definitions and deductive methods which had become customary, contradictions began gradually to appear. These contradictions, the so-called paradoxes of set theory, though at first scattered, became progressively more acute and more serious. In particular, a contradiction discovered by Zermelo and Russell had a downright catastrophic effect when it became known throughout the world of mathematics. Confronted by these paradoxes, Dedekind and Frege completely abandoned their point of view and retreated. Dedekind hesitated a long time before permitting a new edition of his epoch-making treatise Was sind und was sollen die Zahlen to be published. In an epilogue, Frege too had to acknowledge that the direction of his book Grundgesetze der Arithmetik was wrong. Cantor's doctrine, too, was attacked on all sides. So violent was this reaction that even the most ordinary and fruitful concepts and the simplest and most important deductive methods of mathematics were threatened and their employment was on the verge of being declared illicit. The old order had its defenders, of course. Their defensive tactics, however, were too fainthearted and they never formed a united front at the vital spots. Too many different remedies for the paradoxes were offered, and the methods proposed to clarify them were too variegated.
Admittedly, the present state of affairs where we run up against the paradoxes is intolerable. Just think, the definitions and deductive methods which everyone learns, teaches, and uses in mathematics, the paragon of truth and certitude, lead to absurdities! If mathematical thinking is defective, where are we to find truth and certitude?
There is, however, a completely satisfactory way of avoiding the paradoxes without betraying our science. The desires and attitudes which help us find this way and show us what direction to take are these:
Obviously these goals can be attained only after we have fully elucidated the nature of the infinite.
We have already seen that the infinite is nowhere to be found in reality, no matter what experiences, observations, and knowledge are appealed to. Can thought about things be so much different from things? Can thinking processes be so unlike the actual processes of things? In short, can thought be so far removed from reality? Rather is it not clear that, when we think that we have encountered the infinite in some real sense, we have merely been seduced into thinking so by the fact that we often encounter extremely large and extremely small dimensions in reality?
Does material logical deduction somehow deceive us or leave us in the lurch when we apply it to real things or events?1 [Throughout this paper the German word `inhaltlich' has been translated by the words `material' or `materially' which are reserved for that purpose and which are used to refer to matter in the sense of the traditional distinction between matter or content and logical form. -Tr.] No! Material logical deduction is indispensable. It deceives us only when we form arbitrary abstract definitions, especially those which involve infinitely many objects. In such cases we have illegitimately used material logical deduction; i.e., we have not paid sufficient attention to the preconditions necessary for its valid use. In recognizing that there are such preconditions that must be taken into account, we find ourselves in agreement with the philosophers, notably with Kant. Kant taught — and it is an integral part of his doctrine — that mathematics treats a subject matter which is given independently of logic. Mathematics, therefore, can never be grounded solely on logic. Consequently, Frege's and Dedekind's attempts to so ground it were doomed to failure.
As a further precondition for using logical deduction and carrying out logical operations, something must be given in conception, viz., certain extralogical concrete objects which are intuited as directly experienced prior to all thinking. For logical deduction to be certain, we must be able to see every aspect of these objects, and their properties, differences, sequences, and contiguities must be given, together with the objects themselves, as something which cannot be reduced to something else and which requires no reduction. This is the basic philosophy which I find necessary, not just for mathematics, but for all scientific thinking, understanding, and communicating. The subject matter of mathematics is, in accordance with this theory, the concrete symbols themselves whose structure is immediately clear and recognizable.
Consider the nature and methods of ordinary finitary number theory. It can certainly be constructed from numerical structures through intuitive material considerations. But mathematics surely does not consist solely of numerical equations and surely cannot be reduced to them alone. Still one could argue that mathematics is an apparatus which, when applied to integers, always yields correct numerical equations. But in that event we still need to investigate the structure of this apparatus thoroughly enough to make sure that it in fact always yields correct equations. To carry out such an investigation, we have available only the same concrete material finitary methods as were used to derive numerical equations in the construction of number theory. This scientific requirement can in fact be met, i.e., it is possible to obtain in a purely intuitive and finitary way — the way we attain the truths of number theory — the insights which guarantee the validity of the mathematical apparatus.
Let us consider number theory more closely. In number theory we have the numerical symbols
where each numerical symbol is intuitively recognizable by the fact it contains only 1's. These numerical symbols which are themselves our subject matter have no significance in themselves. But we require in addition to these symbols, even in elementary number theory, other symbols which have meaning and which serve to facilitate communication; for example the symbol 2 is used as an abbreviation for the numerical symbol 11, and the numerical symbol 3 as an abbreviation for the numerical symbol 111. Moreover, we use symbols like +, =, and > to communicate statements. 2 + 3 = 3 + 2 is intended to communicate the fact that 2 + 3 and 3 + 2, when abbreviations are taken into account, are the selfsame numerical symbol, viz., the numerical symbol 11111. Similarly 3 > 2 serves to communicate the fact that the symbol 3, i.e., 111, is longer than the symbol 2, i.e., 11; or, in other words, that the latter symbol is a proper part of the former.
We also use the letters a, b, c for communication. Thus b > a communicates the fact that the numerical symbol b is longer than the numerical symbol a. From this point of view, a + b = b + a communicates only the fact that the numerical symbol a + b is the same as b + a. The content of this communication can also be proved through material deduction. Indeed, this kind of intuitive material treatment can take us quite far.
But let me give you an example where this intuitive method is out-stripped. The largest known prime number is (39 digits)
By a well-known method due to Euclid we can give a proof, one which remains entirely within our finitary framework, of the statement that between p + 1 and p! + 1 there exists at least one new prime number. The statement itself conforms perfectly to our finitary approach, for the expression `there exists' serves only to abbreviate the expression: it is certain that p + 1 or p + 2 or p + 3 ... or p! + 1 is a prime number. Furthermore, since it obviously comes down to the same thing to say: there exists a prime number which is
1. >p, and at the same time is
2. £p! + 1,
we are led to formulate a theorem which expresses only a part of what the euclidean theorem expresses; viz., the theorem that there exists a prime number > p. Although this theorem is a much weaker statement in terms of content — it asserts only part of what the euclidean theorem asserts — and although the passage from the euclidean theorem to this one seem quite harmless, that passage nonetheless involves a leap into the transfinite when the partial statement is taken out of context and regarded as an independent statement.
How can this be? Because we have an existential statement, `there exists'! True, we has a similar expression in the euclidean theorem, but there the `there exists' was, as I already mentioned, an abbreviation for: either p + 1 or p + 2 or p + 3 . . . or p! + 1 is a prime number — just as when, instead of saying `either this piece of chalk or this piece or this piece ... or this piece is red' we say briefly `there exists a red piece of chalk among these pieces'. A statement such as `there exists' an object with a certain property in a finite totality conforms perfectly to our finitary approach. But a statement like `either p + 1 or p + 2 or p + 3 ... or (ad infinitum) ... has a certain property' is itself an infinite logical product. Such an extension into the infinite is, unless further explanation and precautions are forthcoming, no more permissible than the extension from finite to infinite products in calculus. Such extensions, accordingly, usually lapse into meaninglessness.
From our finitary point of view, an existential statement of the form `there exists a number with a certain property' has in general only the significance of a partial statement; i.e., it is regarded as part of a more determinate statement. The more precise formulation may, however, be unnecessary for many purposes.
In analyzing an existential statement whose content cannot be expressed by a finite disjunction, we encounter the infinite. Similarly, by negating a general statement, i.e., one which refers to arbitrary numerical symbols, we obtain a transfinite statement. For example, the statement that if a is a numerical symbol, then a + 1 = 1 + a is universally true, is from our finitary perspective incapable of negation. We will see this better if we consider that this statement cannot be interpreted as a conjunction of infinitely many numerical equations by means of `and' but only as a hypothetical judgment which asserts something for the case when a numerical symbol is given.
From our finitary viewpoint, therefore, we cannot argue that an equation like the one just given, where an arbitrary numerical symbol occurs, either holds for every symbol or is disproved by a counter example. Such an argument, being an application of the law of excluded middle, rests on the presupposition that the statement of the universal validity of such an equation is capable of negation.
At any rate, we note the following: if we remain within the domain of finitary statements, as indeed we must, we have as a rule very complicated logical laws. Their complexity becomes unmanageable when the expressions `all' and `there exists' are combined and when they occur in expressions nested within other expressions. In short, the logical laws which Aristotle taught and which men have used ever since they began to think do not hold. We could, of course, develop logical laws which do hold for the domain of finitary statements. But it would do us no good to develop such a logic, for we do not want to give up the use of the simple laws of Aristotelian logic. Furthermore, no one, though he speak with the tongues of angels, could keep people from negating general statements, or from forming partial judgments, or from using tertium non datur. What, then, are we to do?
Let us remember that we are mathematicians and that as mathematicians we have often been in precarious situations from which we have been rescued by the ingenious method of ideal elements. I showed you some illustrious examples of the use of this method at the beginning of this paper. Just as i = (- 1)1/2 was introduced to preserve in simplest form the laws of algebra (for example, the laws about the existence and number of roots of an equation); just as ideal factors were introduced to preserve the simple laws of divisibility for algebraic whole numbers (for example, a common ideal divisor for the numbers 2 and 1 + (- 5)1/2 was introduced, though no such divisor really exists); similarly, to preserve the simple formal rules of ordinary Aristotelian logic, we must supplement the finitary statements with ideal statements. It is quite ironic that the deductive methods which Kronecker so vehemently attacked are the exact counterpart of what Kronecker himself admired so enthusiastically in Kummer's work on number theory which Kronecker extolled as the highest achievement of mathematics.
How do we obtain ideal statements? It is remarkable as well as a favorable and promising fact that to obtain ideal statements, we need only continue in a natural and obvious fashion the development which the theory of the foundations of mathematics has already undergone. Indeed, we should realize that even elementary mathematics goes beyond the standpoint of intuitive number theory. Intuitive, material number theory, as we have been construing it, does not include the method of algebraic computation with letters. Formulas were always used exclusively for communication in intuitive number theory. The letters stood for numerical symbols and an equation communicated the fact that the two symbols coincided. In algebra, on the other hand, we regard expressions containing letters as independent structures which formalize the material theorems of number theory. In place of statements about numerical symbols, we have formulas which are themselves the concrete objects of intuitive study. In place of number-theoretic material proof, we have the derivation of a formula from another formula according to determinate rules.
Hence, as we see even in algebra, a proliferation of finitary objects takes place. Up to now the only objects were numerical symbols like 1, 11, ... , 11111. These alone were the objects of material treatment. But mathematical practice goes further, even in algebra. Indeed, even when from our finitary viewpoint a formula is valid with respect to what it signifies as, for example, the theorem that always
where a and b stand for particular numerical symbols, nevertheless we prefer not to use this form of communication but to replace it instead by the formula
This latter formula is in no wise an immediate communication of something signified but is rather a certain formal structure whose relation to the old finitary statements,
consists in the fact that, when a and b are replaced in the formula by the numerical symbols 2, 3, 5, 7, the individual finitary statements are thereby obtained, i.e., by a proof procedure, albeit a very simple one. We therefore conclude that a, b, =, +, as well as the whole formula a + b = b + a mean nothing in themselves, no more than the numerical symbols meant anything. Still we can derive from that formula other formulas to which we do ascribe meaning, viz., by interpreting them as communications of finitary statements. Generalizing this conclusion, we conceive mathematics to be a stock of two kinds of formulas: first, those to which the meaningful communications of finitary statements correspond; and, secondly, other formulas which signify nothing and which are the ideal structures of our theory.
Now what was our goal? In mathematics, on the one hand, we found finitary statements which contained only numerical symbols, for example,
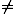 1
1which from our finitary standpoint are immediately intuitable and understandable without recourse to anything else. These statements can be negated, truly or falsely. One can apply Aristotelian logic unrestrictedly to them without taking special precautions. The principle of non-contradiction holds for them; i.e., the negation of one of these statements and the statement itself cannot both be true. Tertium non datur holds for them; i.e., either a statement or its negation is true. To say that a statement is false is equivalent to saying that its negation is true. On the other hand, in addition to these elementary statements which present no problems, we also found more problematic finitary statements; e.g., we found finitary statements that could not be split up into partial statements. Finally, we introduced ideal statements in order that the ordinary laws of logic would hold universally. But since these ideal statements, viz., the formulas, do not mean anything insofar as they do not express finitary statements, logical operations cannot be materially applied to them as they can be to finitary statements. It is, therefore, necessary to formalize the logical operations and the mathematical proofs themselves. This formalization necessitates translating logical relations into formulas. Hence, in addition to mathematical symbols, we must also introduce logical symbols such as
& , V ,  , ~2
(and) (or) (implies) (not)
, ~2
(and) (or) (implies) (not)
[Although Hilbert's original paper used `—' as the sign for negation, we have substituted `~' for greater conformity with the notation used in other papers in this collection. — Eds.]
and in addition to the mathematical variables a,b,c, . . . we must also employ logical variables, viz., the propositional variables A, B, C, . . . .
How can this be done? Fortunately that same preestablished harmony which we have so often observed operative in the history of the development of science, the same preestablished harmony which aided Einstein by giving him the general invariant calculus already fully developed for his gravitational theory, comes also to our aid: we find the logical calculus already worked out in advance. To be sure, the logical calculus was originally developed from an altogether different point of view. The symbols of the logical calculus originally were introduced only in order to communicate. Still it is consistent with our finitary viewpoint to deny any meaning to logical symbols, just as we denied meaning to mathematical symbols, and to declare that the formulas of the logical calculus are ideal statements which mean nothing in themselves. We possess in the logical calculus a symbolic language which can transform mathematical statements into formulas and express logical deduction by means of formal procedures. In exact analogy to the transition from material number theory to formal algebra, we now treat the signs and operation symbols of the logical calculus in abstraction from their meaning. Thus we finally obtain, instead of material mathematical knowledge which is communicated in ordinary language, just a set of formulas containing mathematical and logical symbols which are generated successively, according to determinate rules. Certain of the formulas correspond to mathematical axioms. The rules whereby the formulas are derived from one another correspond to material deduction. Material deduction is thus replaced by a formal procedure governed by rules. The rigorous transition from a naïve to a formal treatment is effected, therefore, both for the axioms (which, though originally viewed naïvely as basic truths, have been long treated in modern axiomatics as mere relations between concepts) and for the logical calculus (which originally was supposed to be merely a different language).
We will now explain briefly how mathematical proofs are formalized. I have already said that certain formulas which serve as building blocks for the formal structure of mathematics are called "axioms." A mathematical proof is a figure which as such must be accessible to our intuition. It consists of deductions made according to the deduction schema
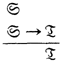
where each premise, i.e., the formulas 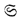 and
and 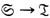 , either
is an axiom, or results from an axiom by substitution, or is the last
formula of a previous deduction, or results from such a formula by
substitution. A formula is said to be provable if it is the last formula
of a proof.
, either
is an axiom, or results from an axiom by substitution, or is the last
formula of a previous deduction, or results from such a formula by
substitution. A formula is said to be provable if it is the last formula
of a proof.
Our program itself guides the choice of axioms for our theory of proof. Notwithstanding a certain amount of arbitrariness in the choice of axioms, as in geometry certain groups of axioms are qualitatively distinguishable. Here are some examples taken from each of these groups:
 (B
(B  A)
A) C)
C)  {(A
{(A  B)
B)
 (A
(A  C)}
C)} (B & ~ B)}
(B & ~ B)}
 ~ A
~ A A
AThe axioms in groups I and II are simply the axioms of the propositional calculus.
 A(b)
A(b) ($a) ~ A(a)
($a) ~ A(a) (a) ~ A(a)
(a) ~ A(a)At this point we discover the very remarkable fact that these transfinite axioms can be derived from a single axiom which contains the gist of the so-called axiom of choice, the most disputed axiom in the literature of mathematics:
 A(eA)
A(eA)where e is the transfinite, logical choice-function.
Then the following specifically mathematical axioms are added to those just given:
 {A(a)
{A(a)
 A(b)},
A(b)},
and finally
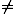 0
0Thus we are now in a position to carry out our theory of proof and to construct the system of provable formulas, i.e., mathematics. But in our general joy over this achievement and in our particular joy over finding that indispensable tool, the logical calculus, already developed without any effort on our part, we must not forget the essential condition of our work. There is just one condition, albeit an absolutely necessary one, connected with the method of ideal elements. That condition is a proof of consistency, for the extension of a domain by the addition of ideal elements is legitimate only if the extension does not cause contradictions to appear in the old, narrower domain, or, in other words, only if the relations that obtain among the old structures when the ideal structures are deleted are always valid in the old domain.
The problem of consistency is easily handled in the present
circumstances. It reduces obviously to proving that from our axioms and
according to the rules we laid down we cannot get `1
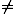 1' as the last formula of a proof, or, in other
words, that `1
1' as the last formula of a proof, or, in other
words, that `1
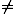 1' is not a provable
formula. This task belongs just as much to the domain of intuitive
treatment as does, for example, the task of finding a proof of the
irrationality of 21/2 in materially constructed
number theory — i.e., a proof that it is impossible to find two
numerical symbols a and b which stand in the relation
a2 = 2b2, or in other words, that
one cannot produce two numerical symbols with a certain property.
Similarly, it is incumbent on us to show that one cannot produce a
certain kind of proof. A formalized proof, like a numerical symbol, is a
concrete and visible object. We can describe it completely. Further, the
requisite property of the last formula; viz., that it read `1
1' is not a provable
formula. This task belongs just as much to the domain of intuitive
treatment as does, for example, the task of finding a proof of the
irrationality of 21/2 in materially constructed
number theory — i.e., a proof that it is impossible to find two
numerical symbols a and b which stand in the relation
a2 = 2b2, or in other words, that
one cannot produce two numerical symbols with a certain property.
Similarly, it is incumbent on us to show that one cannot produce a
certain kind of proof. A formalized proof, like a numerical symbol, is a
concrete and visible object. We can describe it completely. Further, the
requisite property of the last formula; viz., that it read `1
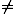 1', is a concretely ascertainable property of the
proof. And since we can, as a matter of fact, prove that it is
impossible to get a proof which has that formula as its last formula, we
thereby justify our introduction of ideal statement.
1', is a concretely ascertainable property of the
proof. And since we can, as a matter of fact, prove that it is
impossible to get a proof which has that formula as its last formula, we
thereby justify our introduction of ideal statement.
It is also a pleasant surprise to discover that, at the very same time, we have resolved a problem which has plagued mathematicians for a long time, viz., the problem of proving the consistency of the axioms of arithmetic. For, wherever the axiomatic method is used, the problem of proving consistency arises. Surely in choosing, understanding, and using rules and axioms we do not want to rely solely on blind faith. In geometry and physical theory, proof of consistency is effected by reducing their consistency to that of the axioms of arithmetic. But obviously we cannot use this method to prove the consistency of arithmetic itself. Since our theory of proof, based on the method of ideal elements, enables us to take this last important step, it forms the necessary keystone of the doctrinal arch of axiomatics. What we have twice experienced, once with the paradoxes of the infinitesimal calculus and once with the paradoxes of set theory, will not be experienced a third time, nor ever again.
The theory of proof which we have here sketched not only is capable of providing a solid basis for the foundations of mathematics but also, I believe, supplies a general method for treatment fundamental mathematical questions which mathematicians heretofore have been unable to handle.
In a sense, mathematics has become a court of arbitration, a supreme tribunal to decide fundamental questions — on a concrete basis on which everyone can agree and where every statement can be controlled.
The assertions of the new so-called "intuitionism" — modest though they may be — must in my opinion first receive their certificate of validity from this tribunal.
An example of the kind of fundamental questions which can be so handled is the thesis that every mathematical problem is solvable. We are all convinced that it really is so. In fact one of the principal attractions of tackling a mathematical problem is that we always hear this cry within us: There is the problem, find the answer; you can find it just by thinking, for there is no ignorabimus in mathematics. Now my theory of proof cannot supply a general method for solving every mathematical problem — there just is no such method. Still the proof (that the assumption that every mathematical problem is solvable is a consistent assumption) falls completely within the scope of our theory.
I will now play my last trump. The acid test of a new theory is its ability to solve problems which, though known for a long time, the theory was not expressly designed to solve. The maxim "By their fruits ye shall know them" applies also to theories. When Cantor discovered his first transfinite numbers, the so-called numbers of the second number class, the question immediately arose, as I already mentioned, whether this transfinite method of counting enables one to count sets known from elsewhere which are not countable in the ordinary sense. The points of an interval figured prominently as such a set. This question — whether the points of an interval, i.e., the real numbers, can be counted by means of the numbers of the table given previously — is the famous continuum problem which Cantor posed but failed to solve. Though some mathematicians have thought that they could dispose of this problem by denying its existence, the following remarks show how wrong they were: The continuum problem is set off from other problems by its uniqueness and inner beauty. Further, it offers the advantage over other problems of combining these two qualities: on the one hand, new methods are required for its solution since the old methods fail to solve it; on the other hand, its solution itself is of the greatest importance because of the results to be obtained.
The theory which I have developed provides a solution of the continuum problem. The proof that every mathematical problem is solvable constitutes the first and most important step toward its solution. . . .3[At this point, Hilbert sketched an attempted solution of the continuum problem. The attempt was, although not devoid of interest, never carried out. We omit it here. — Eds.]
In summary, let us return to our main theme and draw some conclusions from all our thinking about the infinite. Our principal result is that the infinite is nowhere to be found in reality. It neither exists in nature nor provides a legitimate basis for rational thought — a remarkable harmony between being and thought. In contrast to the earlier efforts of Frege and Dedekind, we are convinced that certain intuitive concepts and insights are necessary conditions of scientific knowledge, and logic alone is not sufficient. Operating with the infinite can be made certain only by the finitary.
The role that remains for the infinite to play is solely that of an idea — if one means by an idea, in Kant's terminology, a concept of reason which transcends all experience and which completes the concrete as a totality — that of an idea which we may unhesitatingly trust within the framework erected by our theory.
Lastly, I wish to thank P. Bernays for his intelligent collaboration and valuable help, both technical and editorial, especially with the proof of the continuum theorem.