Resources Math 3 Course Home Page Math 3 Course Syllabus Practice Exams Textbook Home Page Post a Comment

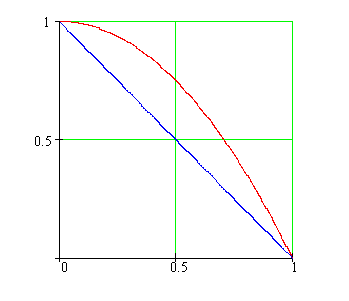
Contents In this lecture we introduce the main ideas of integral calculus; and give an overview of the theme that unifies lectures 21-26, modeling accumulations. Quick Question What is the approximate area under the parabola and above the interval [0,1] that comes from using the triangle instead? Answer Outline Outline for Modeling Accumulations Textbook Modeling Accumulations Today's Homework Log into WebWorK Quiz Modeling Accumulations Quiz Examples Approximate the area of an ellipse using 7 circumscribed rectangles in the first quadrant. Approximate the area of the quarter circle of radius 3 centered at the origin using n circumscribed rectangles, giving your answer in terms of n. The graph of a stream's rate of flow over a 1-hour period is the graph of a sine curve. Assuming the rate of flow is measured every 10 minutes, starting at time 0, use the method of accumulation to aproximate the total volume of flow over this hour, accurate to 3 decimal places. Applets Approximating Areas: Inscribed Polygons Approximating Area: Using Rectangles Accumulation: River Flow Accumulation: Distance Traveled Videos Write 1/5 + 3/25 + 5/125 + 7/625 + ... in sigma notation

Lecture 20 | Index | Lecture 22