Description and Requirements
The Book
Bibliography
Syllabus
 Introduction
Introduction  The Great Pyramid
The Great Pyramid  Music of the Spheres
Music of the Spheres  Number Symbolism
Number Symbolism  Polygons and Tilings
Polygons and Tilings  The Platonic Solids
The Platonic Solids  Roman Architecture
Roman Architecture  Number Symbolism in the Middle Ages
Number Symbolism in the Middle Ages  The Wheel of Fortune
The Wheel of Fortune  Celestial Themes in Art
Celestial Themes in Art  Origins of Perspective
Origins of Perspective  What Shape Frame?
What Shape Frame?  Piero della Francesca
Piero della Francesca  Leonardo
Leonardo  Façade measurement by Trigonometry
Façade measurement by Trigonometry  Early Twentieth Century Art
Early Twentieth Century Art  Dynamic symmetry & The Spiral
Dynamic symmetry & The Spiral  The Geometric Art of M.C. Escher
The Geometric Art of M.C. Escher  Later Twentieth Century Geometry Art
Later Twentieth Century Geometry Art  Art and the Computer
Art and the Computer  Chaos & Fractals
Chaos & Fractals 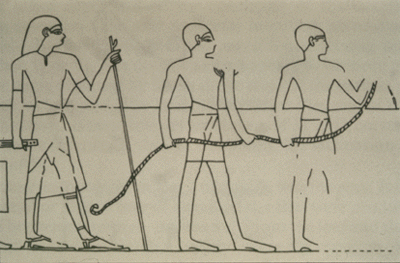
Egyptian Rope Stretchers
Tompkins, Peter. Secrets of the Great Pyramid. NY: Harper, 1971. p. 22.
Welcome to Geometry in Art & Architecture. We'll be taking a long journey together, starting in Egypt, like the travellers in this picture. There, the story goes, geometry got its start when rope stretchers were sent out to put back the boundary markers washed away by the Nile.
In addition to looking at art and architecture, we'll cover any mathematics-related topics as we go along. The Math Topics for the first unit will be an introduction to the triangle in general, and the so-called Egyptian triangle, contained in the great pyramid. Since the Egyptian triangle contains the golden ratio, we'll introduce the ideas of ratio and proportion here, and for squaring of the circle, we must be able to find perimeters and areas of the square and the circle.
The plan is to go more or less chronologically, following threads of Art, Mathematics, and Architecture, from Egypt to the present.
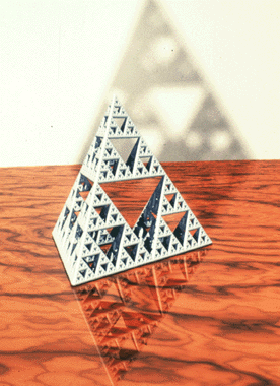 |
Fractal Tetrahedron |
We'll start our journey with a pyramid, and we'll also end with a very different pyramid, a Sierpinski tetrahedron, in our final unit on Chaos and Fractals.
We'll limit ourselves to Western art only, but even with that restriction the coverage is very wide. That means, of course, that we can't go too deeply into any one topic.
Things We'll Look For
 |
Brunes, Tons, The Secrets of Ancient Geometry - and its use.
Copenhagen. Rhodos, 1967.
Brune's Cover |
As we go through the material we'll be looking for:
- Proportions between the parts of a building, a painting, or a sculpture. In particular, we'll look for the golden ratio and the musical ratios.
- Use of geometric symbols, such as the circle, mandala, triangle, square, pentagram, hexagon, or octagon, and their use in so-called Sacred Geometry.
- Geometric Constructions, like squaring the circle, the Gothic Master Diagram, the sacred cut, and constructions of the pentagon.
- Shapes of Frames; how they are chosen and how they affect the contents of a painting.
- Art Motifs, especially recurring themes that we see over and over in art.
- Math content; any geometry or other math that is closely related to the art or architecture we're studying.
- People, ones that played a key role in developing the ideas related to this course, and especially those that were both mathematicians and artists or architects.
Skeptical Attitude
We'll see that writers in this field sometimes make unsupported claims. Rudolf Wittkower, in his Architecture in the Age of Humanism says
". . . in trying to prove that a system of proportions has been deliberately applied . . . one is easily misled into finding . . . those ratios which one sets out to find. Compasses in the scholar's hand do not revolt."
In other words, we tend to find what we're looking for, whether its there or not. We will hope to avoid that pitfall by questioning everything.
Mathematics Across the Curriculum
MATC Logo
This course is one of several developed under a grant from the National Science Foundation to Dartmouth, called Mathematics Across the Curriculum. Some courses being developed at Dartmouth are ones that try to integrate math with:
| physics and chemistry |
| textile design |
| psychology and medicine |
| music |
| earth sciences |
| Renaissance thought |
and this one, combining math with art and architecture.
The Eternal Golden Braid
 |
Hofstadter, Douglas. Gödel, Escher, Bach: an Eternal Golden Braid. NY:
Vintage, 1979. Hofstadter Cover |
In "Gödel, Escher, Bach:", Douglas Hostadter says
"I have sought to weave an eternal golden braid out of these three strands, Gödel, Escher, Bach, a mathematician, an artist, and a composer."
In other words, math, art, and music. In this course we hope to trace just two strands of his eternal golden braid, art (and architecture) and math, and sometimes connect them with strands from literature, mythology, and religion .
We've planned an exciting journey, to follow these strands over 5000 years and several continents, and we really hope that you'll join us for the trip!
