Polygons, Tilings,
&
Sacred Geometry

Slide 5-1: Pompeii pavement
Calter photo

In the last unit, Number Symbolism, we saw that in the ancient world certain numbers had symbolic meaning, aside from their ordinary use for counting or calculating.

In this unit we'll show that the plane figures, the polygons, triangles, squares, hexagons, and so forth, were related to the numbers (three and the triangle, for example), were thought of in a similar way, and in fact, carried even more emotional baggage than the numbers themselves, because they were visual. This takes us into the realm of Sacred Geometry.

For now we'll do the polygons directly related to the Pythagoreans; the equilateral triangle (Sacred tetractys), hexagon, triangular numbers, and pentagram. We'll also introduce tilings, the art of covering a plane surface with polygons.

Outline:	Polygons
	EquilateralTriangle
	Tilings
	Hexagon & Hexagram
	Pentagon & Pentagram
	Golden Triangle
	Conclusion
Reading
Projects

Slide 5-23: Design at Pompeii

Calter photo

In the last unit, Number Symbolism we saw that in the ancient world certain numbers had symbolic meaning, aside from their ordinary use for counting or calculating. But each number can be associated with a plane figure, or polygon (Three and the Triangle, for example).

In this unit we'll see that each of these polygons also had symbolic meaning and appear in art motifs and architectural details, and some can be classified as sacred geometry.

A polygon is a plane figure bounded by straight lines, called the sides of the polygon.

From the Greek poly = many and gon = angle

The sides intersect at points called the vertices. The angle between two sides is called an interior angle or vertex angle.

Regular Polygons

A regular polygon is one in which all the sides and interior angles are equal.

Polygons vs. Polygrams

A polygram can be drawn by connecting the vertices of a polgon. Pentagon & Pentagram, hexagon & hexagram, octagon & octograms

Slide 5-2: Tablet in School of Athens, showing Tetractys Bouleau

There are, of course, an infinite number of regular polygons, but we'll just discuss those with sides from three to eight. In this unit we'll cover just those with 3, 5, and 6 sides. We'll start with the simplest of all regular polygons, the equilateral triangle.

Sacred Tetractys

The Pythagoreans were particularly interested in this polygon because each triangular number forms an equilateral triangle. One special triangular number is the triangular number for what they called the decad, or ten, the sacred tetractys.

Ten is important because it is, of course, the number of fingers. The tetractys became a symbol of the Pythagorean brotherhood. We've seen it before in the School of Athens.

Trianglular Architectural Features

Slide 8-11: Church window in Quebec

In architecture, triangular windows are common in churches, perhaps representing the trinity.

Other three-branched or three-comered designs include the triskelion.

Slide 5-3: Greek Triskelion: Victory and Progress
Lehner, Ernst. Symbols, Signs & Signets. NY: Dover, 1950 p. 85

Slide 5-4: Irish Triskelions from Book of Durrow.
Met. Museum of Art. Treasures of Early Irish Art. NY: Met. 1977

Slide 5-5: Calter carving Mandala II Calter photo

Slide 5-6: Closeup of wheel Calter photo

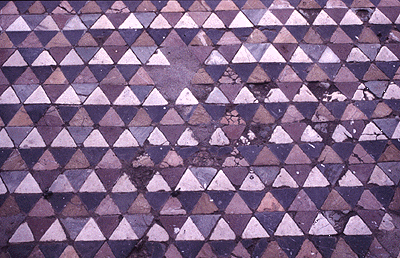Slide 5-7: Pompeii Tiling with equilateral triangles Calter photo

Tilings or tesselations refers to the complete covering of a plane surface by tiles. There are all sorts of tilings, some of which we'll cover later. For now, lets do the simplest kind, called a regular tiling, that is, tiling with regular polygons.

This is opposed to semiregular tilings like the Getty pavement shown here.

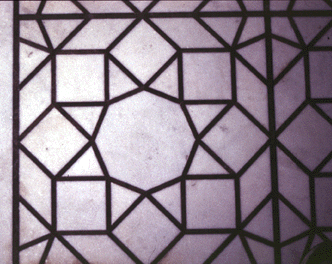Slide 5-8: Getty Pavement Calter photo

The equilateral triangle is one of the three regular polygons that tile a plane. the other two being the square and hexagon.

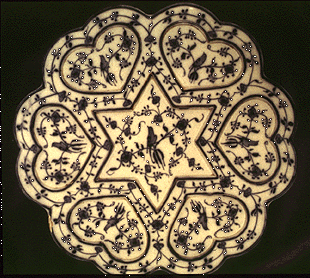Slide 5-15: Plate with Star of David Keller, Sharon. The Jews: A Treasury of Art and Literature. NY: Levin Assoc. 1992

Hexagonal Tilings

Our next polygon is the hexagon, closely related to the equilateral triangle

The hexagon is a favorite shape for tilings, as in these Islamic designs, which are not regular tilings, because they use more than one shape.

Slide 5-9: Islamic Tiling Patterns
El-Said, Issam, et al. Geometric Concepts in Islamic Art. Palo Alto: Seymour, 1976. p. 54

An Illusion

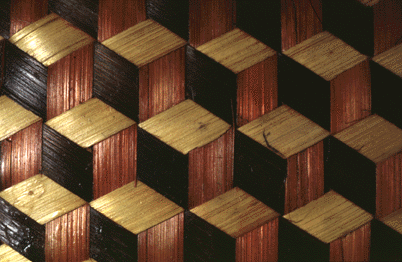Slide 5-10: Basket Calter photo

Slide 5-11: Pavement, Ducal Palace, Mantua Calter photo

The Hexagon in Nature

The hexagon is found in nature in the honeycomb, and some crystals such as basalt, and of course, in snowflakes.

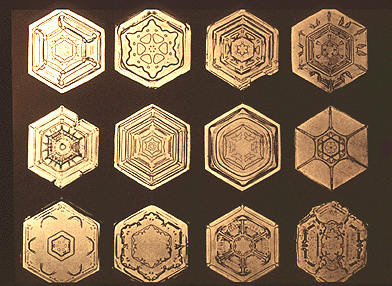Slide 5-12: Snowflakes Bentley, W. A. Snow Crystals. NY: Dover, 1962.

Six-Petalled Rose

The hexagon is popular in architectural decoration partly because it is so easy to draw. In fact, these are rusty-compass constructions, which could have been made with a forked stick.

Six circles will fit around a seventh, of the same diameter, dividing the circumference into 6 equal parts, and the radius of a circle exactly divides the circumference into six parts, giving a six petalled rose.

Slide 5-13: Moses Cupola. S. Marco, Venice Demus, Otto. The Mosaic Decoration of San Marco, Venice. Chicago: U. Chicago, 1988. plate 60.

Hexagon vs. Hexagram

Connecting alternate points of a hexagon gives a hexagram, a six-pointed star, usually called the Star of David, found in the flag of Israel.

Slide 5-14: Star of David on Silver bowl from Damascus.
Jewish Museum (New York, N.Y.), Treasures of the Jewish Museum. NY: Universe, 1986. p. 61

Solomon's Seal

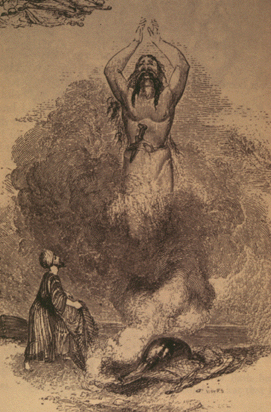Slide 5-17: The genii emerging. Burton, Richard. The Arabian nights entertainments. Ipswich : Limited Editions Club, 1954.

The U.S. Great Seal

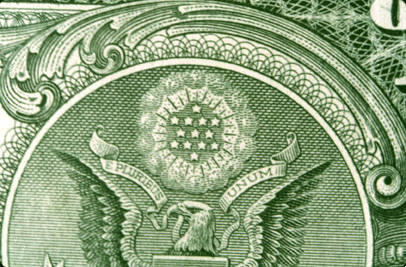Slide 5-20: Seal on Dollar Bill Calter photo

The hexagrarn can also be viewed as two overlapping Pythagorean tetractys.

Joseph Campbell writes; In the Great Seal of the U.S. there are two of these interlocking triangles. We have thirteen points, for our original thirteen states, and six apexes: one above, one below, andfour to thefour quarters. The sense of this might be thalftom above or below, orftom any point of the compass, the creative word may be heard, which is the great thesis of democracy.
- The Power of Myth. p.27

Hexagonal Designs in Architecture

Hexagonal designs are common in ancient architecture, such as this church window in Quebec.

Slide 5-22: Church Window in Quebec Calter photo

This marvelous design is at Pompeii. It is made up of a central hexagon surrounded by squares, equilateral triangles, and rhombi.

Slide: 5-23. Design at Pompeii
Calter photo

Slide 5-24: Design on Pisa Duomo
Calter photo

This hexagram is one of countless designs on the Duomo in Pisa.

Slide 5-26: Pentagram from grave marker Calter photo

The Pentagram was used as used as a sign of salutaton by the Pythagoreans, its construction supposed to have been a jealously guarded secret. Hippocrates of Chios is reported to have been kicked out of the group for having divulged the construction of the pentagram.

The pentagram is also called the Pentalpha, for it can be thought of as constructed of five A's.

Euclid's Constructions of the Pentagon

Euclid gives two constructions in Book IV, as Propositions 11 & 12. According to the translator T.L. Heath, these methods were probably developed by the Pythagoreans.

Medieval Method of Construction

Supposedly this construction was one of the secrets of Medieval Mason's guilds. It can be found in Bouleau p. 64.

Durer's Construction of the Pentagon

Another method of construction is given in Duret's "Instruction in the Measurement with the Compass and Ruler of Lines, Surfaces and Solids," 1525.

Its the same construction as given in Geometria Deutsch, a German book of applied geometry for stonemasons and

Golden Ratios in the Pentagram and Pentagon

The pentagon and pentagram are also interesting because they are loaded with Golden ratios, as shown in Boles p.48.

Slide 5-28: Emmer, plate F3
Emmer, Michele, Ed. The Visual Mind: Art and Mathematics. Cambridge: MIT Press, 1993.

The Golden Triangle

A golden triangle

also called the sublime triangle, is an isoceles triangle whose ratio of leg to base is the golden ratio.

It is also an isoceles triangle whose ratio of base to leg is the golden ratio, so there are two types: Type I, acute, and type II, obtuse.

A pentagon can be subdivided into two obtuse and one acute golden triangle.

Euclid's Construction

Euclid shows how to construct a golden triangle. Book IV, Proposition 10 states, "To construct an isoceles triangle having each of the angles at the base the double of the remaining one."

Penrose Tilings

Slide: 5-27: Penrose Tilings. Kappraff, Jay. Connections: The Geometric Bridge between Art & Science. NY: McGraw, 1990. p. 195

One place that the golden triangle appears is in the Penrose Tiling, invented by Roger Penrose, in the late seventies. The curious thing about these tilings is they use only two kinds of tiles, and will tile a plane without repeating the pattern.

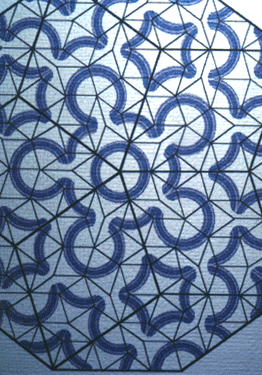Slide 5-29: NCTM Cover

So we covered the triangle, pentagon, and hexagon, with sides 3, 5, and 6. We'll cover the square and octagon in a later unit.

Its clear that these figures, being visual, carried even more powerful emotional baggage than the numbers they represent.

Next time we'll again talk about polygons, in particular the triangle. But I won't waste your time with some insignificant and trivial fact about the triangle, but will show that, according to Plato, triangles form the basic building block of the entire universe!

Reading

Joseph Campbell, The Power of Myth, pp. 25-29
Carl Jung, Man andHis Symbols, pp. 266-285
Euclid, Elements, V2, pp. 97-104
Kappraff, Connections, pp. 85-87, 195-197
Fisher, p. 92-94

Cut a circle from paper, fold in quarters vertically, then again horizontally, making a 4 x 4 grid.
Mark the circumference where it crosses the grid. Connect these points in various ways to make the familiar regular polygons.	2
All these figures can be folded see Magnus Wenninger,Mathematics Through Paper Folding Fold an equilateral triangle using NCTM method	4
Construct a hexagon with compass	8
Construct a hexagon by paper folding, NCTM method	8
Construct a hexagon by folding a circle	8
Make a pentagram by extending the sides of a pentagon, or make a pentagram by connecting the vertices of a pentagon	12
Construct a pentagon by either of Euclid's methods. Connect the vertices to make a pentagram.	13
Construct a pentagon by the Medieval method. Connect the vertices to make a pentagram	14
Construct a pentagon by Durer's method	14
Check for in the pentagon by using dividers	14
Solve the five-disk problem, Huntley p. 45	14
Put one type I and two type 11 golden triangles together to form a pentagon	15
Construct a triangle by Euclid's method	16
Construct a kite and a dart. Make xerox copies. Use them to make a Penrose tiling.	17