

 Lesson 5 Art Lecture part 2
Lesson 5 Art Lecture part 2
We will now look at some mathematical properties of tessellations that can aid us in understanding the circular geometry of Islamic tessellations.
Properties of polygons and Tesselations
The following discussion is paraphrased from Seymour, Dale and Britton, Jill. Introduction to Tesselations.
The word tessellation comes from the Latin tessella, a the small square stone or tile used in ancient Roman mosaics." Tilings and mosaics are common synonyms for tesselations. A plane tessellation is a pattern made up of one or more shapes, completely covering a surface without any gaps or overlaps. Some shapes tessellate without overlapping or leaving spaces and some do not... When we analyze a tessellation pattern, we are interested in the closed figure, or tiles, that form the overall design. The simplest class of closed figures are polygons. Circles are polygons, and squares and triangles, etc. are all closed figures that can be inscribed within a circle. Each has a perimeter and an area. Polygons are formed by line segments, that meet at their endpoints and are named according to the number of sides and angles they contain. Because of their symmetrical character we will be working with regular polygons: pentagons, hexagons, etc. A regular polygon is one with all its angles and sides congruent. The more sides a regular polygon has, the greater its angles. "Observe that as the number of sides in the regular polygon increases, the closer it comes to appearing like a circle. In addition, the circle encases every conceivable symmetry?
How will this affect the patterns?
Triangles are the simplest regular polygons with the most acute angles: 60 degrees. The angles of any triangle add up to = 180. Triangles, rectangles and hexagons are the only regular polygons that tile the plane by themselves. When hexagons fill the space around a point, three equal angles meet at a common vertex point and the sum of their angles divides evenly into the 360 degrees of a circle. This is true of the angles of triangles and squares, but not pentagons. Polygons larger than hexagons cannot tile the plane because they leave gaps or overlap. This is true of pentagons as well. Accordingly, when polygons larger than hexagons fill the space around a common vertex point, the sum of their angles does not divide evenly into 360. Since the polygon angles get larger as the number of sides increases, the next time we could fill the 360 with equal angles would be when we had two angles measuring 180 degrees. This is a straight line and no longer a polygon. Therefore, unless we are using triangles, rectangles or hexagons, we will have to use more than one regular polygon whose angles add up to a sum that can be evenly divided into by 360 in order to tile a plane without gaps or overlaps. Page 45
From this it is apparent that the circle and its symmetrical division are the basic feature of Islamic tiling.
The following discussion is quoted from a paper, written by Dartmouth student, Susannah McFaddan in June,1995.
The circle and its center are the point at which all Islamic patterns begin. Any regular polygon can be drawn within it by conecting points equidistant to each other on the circle's edge. However the circle must be repeated in order to create patterns.
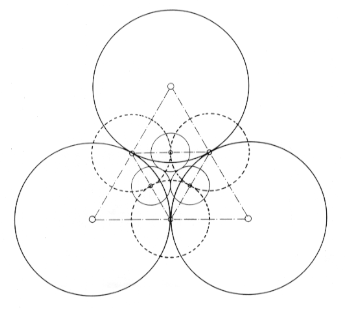
When identical copies of a circle are placed together with their outermost edge touching so that they retain their separate identity while also becoming part of a larger shape, their centers rather than their edges become the point at which lines are connected. When the centers of three united circles are joined by lines, an equilteral triangle is created as shown in figure 1.

Another interesting feature of this is figure is when the points on the edges of the circles (where they touch the other circles) are connected by lines, the lines also form an equilateral triangle within the larger triangle. The triangle is the simplest figure to which all other polygons can be reduced;
Yet the symmetry of this figure is very complex. If three circles with half the radius of the original circles are drawn around the points at which the original circles touch the others, an exact replication of the original figure is created. This repetition can repeat infinitely as shown in Figure 2.
[From this diagram we can also also see the harmonious proportions derived from the construction of nested polygons. In each square repeat unit (Figure 12a) the proportion of the side of the inscribed quare ACBD to the side of the circumscribed square is abcd is AD = 1 (i.e., ad = AB, the diagonal of the inscribed square). Thus the sides of the seris of concentric squres drawn in Figure 12 be are related by proportion 1: 2, and therefore the areas are progressively halved.]
Illustration. Page 8 concepts.
As more circles are added to this figure, larger polygons are created as illustrated in Figure 3.
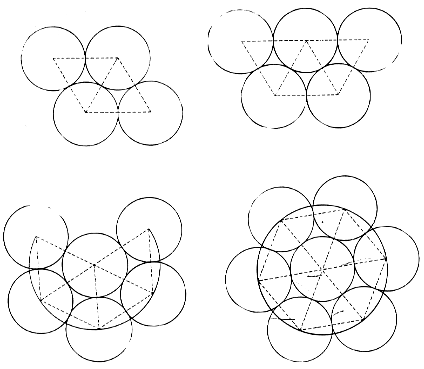
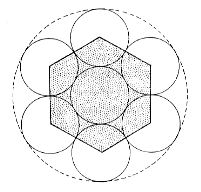
The most important configuration of circles arises when six circles with equal radiaes ar arranged around a seventh circle also with equal areas so that the edge of each circle on the outside is touching the edge of the inner cicle as well as the ones beside it. Figure 3c. From this configuration the three most important polygons to Islamic art: the triangle, the square and the hexagon, can be formed.
By either connecting the centers of thecircles with liens or by drawing tangents through the points at which the circles connect.

The equilateral triangle, the hexagon and the square are the three shapes that can tessellate and grow without leaving any gaps.
They repesent an infinitely small and large growth system.
The principles of unity, abstraction and harmony with nature and the heavens,
as fundamental to Islamic art, are symbolized in this circular geometry of the plane.
The square and the rectangle have harmonious proportions, and the circle is
the ultimate epxression of unity. The circle is not only the perfect expression
of justice-equality in all directions in a finite domain--but also the most
beautiful parent of all polygons, both containing and underlying them.
From the circle comes the triangle, square and hexagon. These three shapes are
thus meant to symbolize "the square of the earth, or materiality, the triangle
of human consiousness, and the hexagon, or circle of heaven.
Critchlaw
Bibliography
Allen, Terry. Five Essays on Islamic Art. Solipsist Press, 1988.Abas, Syed Jan and Salman, Amer Shaker. Symmetries of Islamic Geometrical Patterns. Singapore, New Jersey, London, Hong Kong: World Scientific, 1995.
Burckhardt, Titus. Art of Islam; Language and Meaning. World of Islam Publishing Company Ltd., 1976
Grabar, Oleg. Islamic art and architecture.
Haneef, Suzanne. What everyone should know about Islam and Muslims. Des Plaines, IL: Library of Islam, 1985.
Seymour, Dale and Britton Jill. Introduction to Tesselations. Palo Alto, CA: Dale Seymour Pubications,1989.
El-Said, Issam and Parman, Ayse. Geometric Concepts in Islamic Art. Palo Alto, CA: Dale Seymour Pubications,1976.
Critchlow, Keith. Islamic Pattern: An Analytical and Cosmological Approach. Schocken books, New, York, NY. 1976.


El-Said and Parman: "Geometric Concepts in Islamic Art",
p. 114
6 Artistic Crea1ivity and the Geometric Method of Design
Geometric patterns are constructed by the recurrence of geometrical forms, the resultant ordering of which is determined by the creative act. When the systems of proportioning have been selected, the creation of patterns by infinite combinations of the geometric forms providing these systems of proportioning becomes possible. The designer thus has the freedom of choosing his systems of construction as well as the freedom of constituting the variations made possible by these systems. The geometric method therefore establishes an approach to art in which the execution and the aesthetics of the composition is systematically determined. However, it cannot be regarded as a purely mechanical process on account of its capacity to accommodate human self-expression. The reader must now conceive that the ability to exploit the freedom of expression by the geometric method necessitated a deep familiarity with the available systems of construction only acquired after long periods of experience. The knowledge gained in this field, being very broad and detailed, was only transmitted through apprenticeship. The artistic achievement, as in music, was an abstract or intellectual beauty arising from order or 'truth', with expressiveness and originality based on the depth of knowledge and skill, in that order, the joyful pursuit of the usually 'anonymous' artist who thus wove his identitv into his work.
References
Bourgoin, J. and Hankin, E. H.
 Lesson 1 | Math part
| Art part |
Lesson 1 | Math part
| Art part |