Skip to main content

Section 1.2 Paradoxes about infinities and infinite divisions

Worksheet
A4US

Let's think about an old philosophical argument. Figure Figure 1.2.1 shows a heap of sand - a pile of individual grains. Suppose we take a gain of sand away from the heap. Is it still a heap? I think most of us would say that it is. So, take another grain away. Is it still a heap? Probably.

Figure 1.2.1. A heap of sand.

If we continue this rather tedious removal of one grain at a time, at some point in the distant future we will be left with a single grain of sand in our heap. Is it still a heap? Here, I think most people would say no. So somewhere in this process, the heap ceased to be a heap. But at what point did this transition happen?

The word sorites means "heap" in Greek - like a heap of sand, a heap of laundary, or a heap of trouble. It has also come to represent the type of argument we just made about the pile of sand. There are lots of arguments like this we can make, many of them about mathematical topics. In this set of exercises, we will explore several of these.

1. Heaps.

As we think about the process of removing sand from a heap a grain at a time, talk with your group about the last question - when does the heap cease to be a heap? Why do you think this is a hard question to answer?

2. Line segments.

For the sake of concreteness, we'll think about the specific line segment \([0,1]\) on the usual number line as depicted in Figure Figure 1.2.2. If we divide the line segment in half, is the result two line segments? What about if we divide them again? Are they still line segments? If we keep dividing and dividing, is the result ever not a line segment?

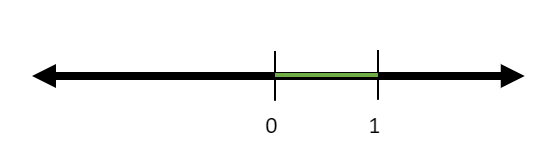
Figure 1.2.2. The interval \([0,1]\) on the real number line.

On the other hand, we can think of \([0,1]\) as the collection of all the points on the line, when labeled by their coordinate, lie between zero and one (inclusive). How do you think these two points of view interact with one another?

3. Accumulation and area.

That which has no thickness cannot be piled up; yet it is a thousand 里 1  in dimension.

里 (li) is a unit of measurement used in the traditional Chinese system. One 里 is about a third of a mile or half a kilometer.

The quote above is attributed to Hui Shi, a Chinese philosopher who lived in the third centruy BCE who, like the Greek philosopher Zeno, was famous for his paradoxes.

This paradox illustrates a conundrum similar to the previous exercise but in reverse. In Figure 1.2.3 we see a kind of lumpy region shaded in grey. We can think of the area as made up of lines and visualize it by sweeping a line from the top of the figure to the bottom - you can visualize this by player the video in the figure. A line segment is a one-dimensional object - it has length but no width. But, as Shi Hui observes, "piling up" infinitely many of them somehow creates an extra dimension.

Figure 1.2.3. A video of a line sweeping out the area of a grey lumpy region.

In your group, discuss this paradox. How do you think it is possible to aggregate one dimensional objects into two dimensional area?